
In retrospect, the combination of these steps is something that anyone could have come up with, which makes it more surprising that this method is not commonly known. It is also called an Equation of Degree 2 (because of the 2.

I found that the individual steps of this method had been separately discovered by ancient mathematicians, and some deep digging unearthed modern teachers who were on the same track. The name Quadratic comes from quad meaning square, because the variable gets squared (like x2). I publicly shared a formal article, while continuing to investigate mathematical history around quadratic equations. Adding this technique as a standard method would directly improve the learning experience for anyone trying to understand this topic, which is part of the regular mathematical curriculum everywhere in the world. I was very surprised, as this method was easier to understand than what is typically written in textbooks. One night in September, while brainstorming different ways to think about the quadratic formula, I came up with a simple way to solve quadratic equations that I had never seen before.
#Quadratic equations how to
Note that the quadratic formula actually has many real-world applications, such as calculating areas, projectile trajectories, and speed, among others.I've recently been thinking about how to explain school math concepts in more thoughtful and interesting ways, while creating my Daily Challenge lessons. This is demonstrated by the graph provided below. Furthermore, the quadratic formula also provides the axis of symmetry of the parabola. The x values found through the quadratic formula are roots of the quadratic equation that represent the x values where any parabola crosses the x-axis. i.e., when each of them is substituted in the given equation we get 0. They are also known as the 'solutions' or 'zeros' of the quadratic equation.For example, the roots of the quadratic equation x 2 - 7x + 10 0 are x 2 and x 5 because they satisfy the equation.
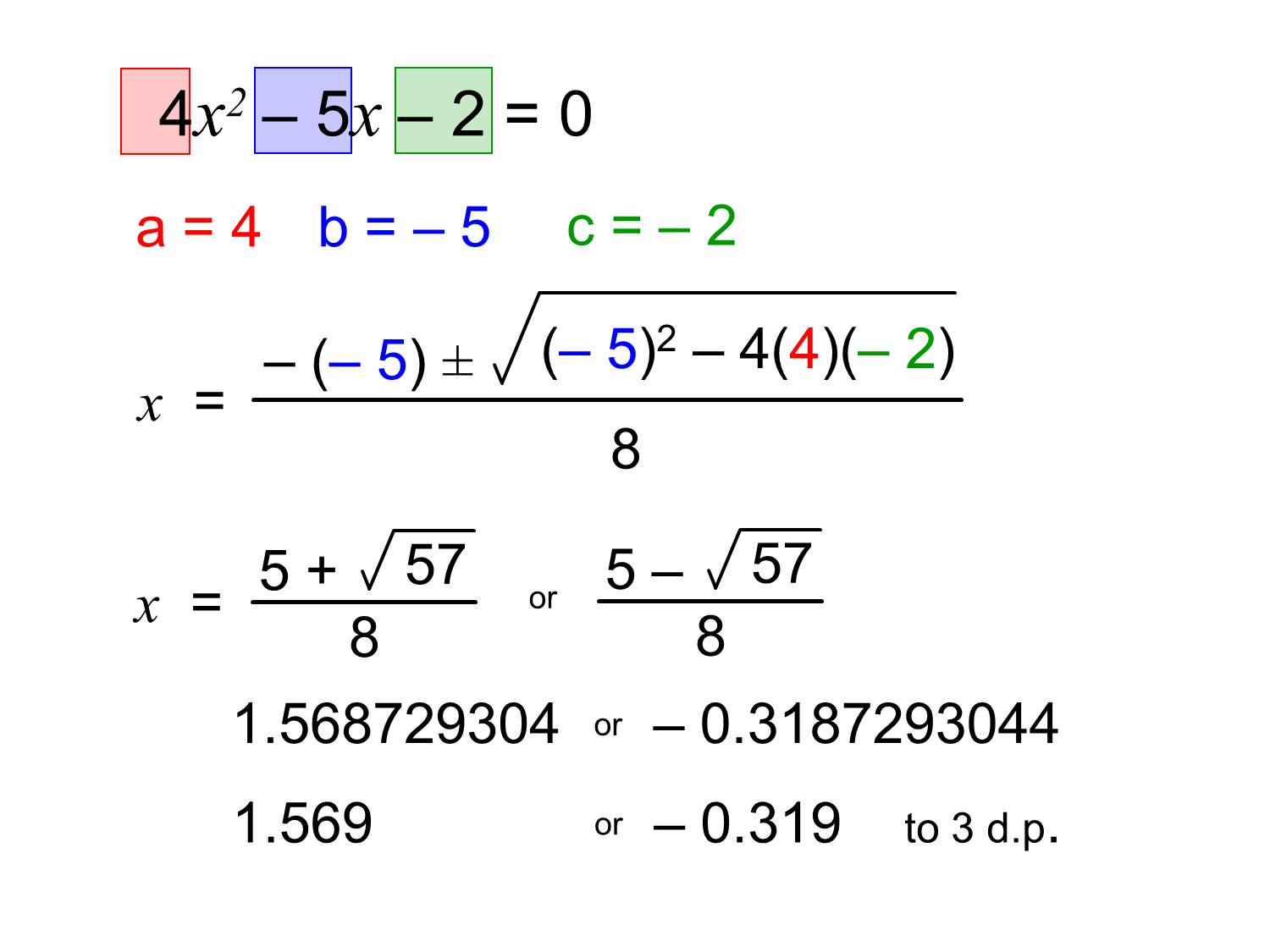
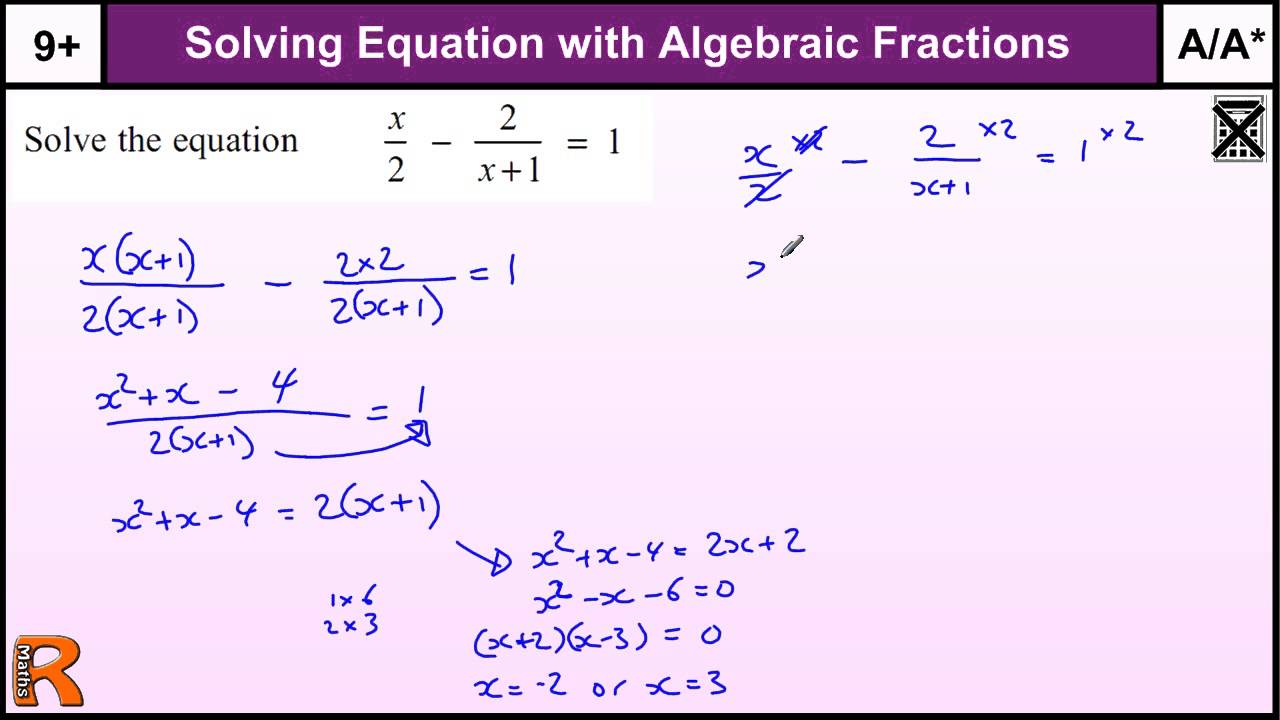
Recall that the ± exists as a function of computing a square root, making both positive and negative roots solutions of the quadratic equation. The roots of a quadratic equation are the values of the variable that satisfy the equation. Below is the quadratic formula, as well as its derivation.įrom this point, it is possible to complete the square using the relationship that:Ĭontinuing the derivation using this relationship: Only the use of the quadratic formula, as well as the basics of completing the square, will be discussed here (since the derivation of the formula involves completing the square). A quadratic equation can be solved in multiple ways, including factoring, using the quadratic formula, completing the square, or graphing. For example, a cannot be 0, or the equation would be linear rather than quadratic. The numerals a, b, and c are coefficients of the equation, and they represent known numbers. Here we will learn about the quadratic equation and how to solve quadratic equations using four methods: factorisation, using the quadratic equation formula, completing the square and using a graph. Where x is an unknown, a is referred to as the quadratic coefficient, b the linear coefficient, and c the constant. In algebra, a quadratic equation is any polynomial equation of the second degree with the following form: Fractional values such as 3/4 can be used.


 0 kommentar(er)
0 kommentar(er)
